|
This is a file from the Wikimedia Commons. Information from its description page there is shown below.
Commons is a freely licensed media file repository. You can help.
|
Summary
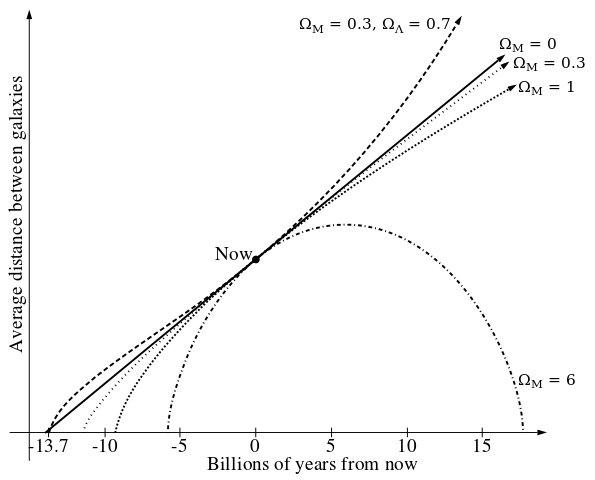
| DescriptionFriedmann universes.svg |
Intended as a replacement for File:Universe.svg and File:Universos.gif. Improvements: better dash patterns, more accurate curves (actual solutions of the Friedmann equations, not hand-drawn).
|
| Date |
23 September 2009 |
| Source |
Own work |
| Author |
BenRG |
Permission
( Reusing this file) |
Public domain
|
Formulas
This diagram uses the following exact solutions to the Friedmann equations:

See also
Some of the shown models are implemented as an animation at Cosmos-animation.
Licensing
| Public domainPublic domainfalsefalse |
 |
I, the copyright holder of this work, release this work into the public domain. This applies worldwide.
In some countries this may not be legally possible; if so:
I grant anyone the right to use this work for any purpose, without any conditions, unless such conditions are required by law.Public domainPublic domainfalsefalse
|
 The categories of this image should be checked. Check them now!
The categories of this image should be checked. Check them now!
- Remove redundant categories and try to put this image in the most specific category/categories
- Remove this template by clicking here (or on the first line)
File usage on other wikis
Related galleries
File usage
The following pages on Schools Wikipedia link to this image (list may be incomplete):
This file contains additional information, probably added from the digital camera or scanner used to create or digitize it. If the file has been modified from its original state, some details may not fully reflect the modified file.
Through Schools Wikipedia, SOS Children's Villages has brought learning to children around the world. SOS Children's Villages helps more than 2 million people across 133 countries around the world. Would you like to sponsor a child?